Blackjack card counting software. Award winning casino practice software tools for beatable games: Blackjack card counting & shuffle tracking. The major aim in the construction of these tools is the accurate simulation of play in a casino. In professional blackjack there is about 150 for Hi-lo full as far as I remember since reading the 9 years ago. However, in Professional blackjack the Hi-lo is modified by Wong. It might not contain the traditional indices of the system. Since the traditional system is first created by Harvey Dubner in 1963. Blackjack Science's mission is to teach how to legally extract funds from gaming establishments by gaining a mathematic advantage and developing the skills required to use that advantage. The scope and quality of products and services are designed to serve everyone from the recreational player to the seasoned advantage player. Let us mentor you to your desired level of proficiency. Winning tactics in Blackjack require that the player play each hand in the optimum way, and such strategy always takes into account what the dealer's upcard is. When the dealer's upcard is a good one, a 7, 8, 9, 10-card, or ace for example, the player should not stop drawing until a total of 17 or more is reached.
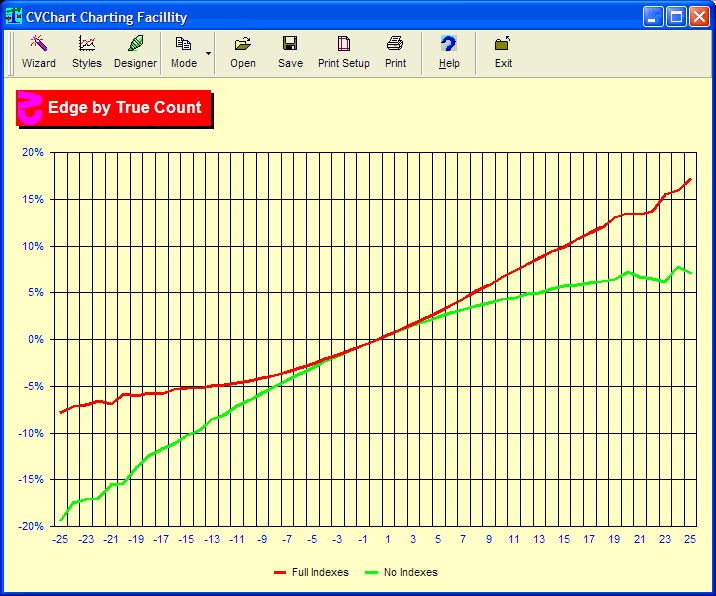
- Blackjack Full Indices Definition
- Blackjack Full Indices Meaning
- Blackjack Full Indices 2020
- Full Indexes Blackjack
- Blackjack Full Indices Vs
Yea I was thinking that due to the increased accuracy of playing efficiency, it might lead to smaller variance because there would be less playing mistakes. For betting correlation I keep a side count of aces dealt & add or subtract 2 to the running count based on aces dealt vs aces that should have been dealt. Apparently this increases the betting correlation of AOII to .99%. I believe the accuracy of playing efficiency & insurance correlation lowers the house edge off the top. I read that in a 6 deck game the Hi-Lo Ill 18 basic strategy variations decreases the house edge by 0.2% but in a double deck game playing efficiency is much more important. I wonder if using the AOII with over 50 basic strategy variations in a double deck S17 DAS game with an off the top house edge of 0.27% reduces the house edge so much as to create an off the top player advantage?? Would love to see a simulation of this.
The Hi-Lo Lite (Or, Why All Those Index Numbers You Learned Never Really Mattered)
By Arnold Snyder
 (From Blackjack Forum Vol. XI #3, September 1991)
(From Blackjack Forum Vol. XI #3, September 1991)© 1991, 2005 Arnold Snyder
Last June, I started writing a monthly blackjack card counting column for Casino Player magazine, which comes out of Atlantic City. It’s a Q/A column.
One of the recent questions that Casino Player’s editors forwarded to me, which took me three issues to answer, has resulted in my development of a new approach to card counting—a system I call the Hi-Lo Lite. This system would be ideal for any player who feels the Red Seven Count is too simplified, with too much of a power loss in single and double-deck games.
In my Casino Player articles, I described my method of developing the Hi-Lo Lite system, so that a knowledgeable card counter could easily convert the Hi-Lo (or any balanced count) to a powerful 'lite' version. And I compared the power of my multiple-deck lite indices with the power of Stanford Wong’s full set of indices (from Professional Blackjack).
But I didn’t publish the one-deck system, since Casino Player is aimed at more casual players, many of whom would require lengthy descriptions of the meaning of 'strategy index numbers,' 'true count,' etc.
Here in Blackjack Forum, for the first time ever, is my complete Hi-Lo Lite system. For those new to counting, the Hi-Lo Count card values are 2 to 6 = +1; 10,A = -1; and 7, 8, 9 = 0. In this article, I assume that you understand how to use strategy indices, convert running count to true count (per deck), etc.
How Much Power Do You Lose With Simplified Indices? The Sims Say None.
First, some background information…
The initial question from the Casino Player reader that sparked the development of this new approach to card counting was: 'Which Hi-Lo strategy indice tables are more accurate—Stanford Wong’s or Julian Braun’s?'
This was a question that I didn’t know the answer to, but which I felt would be fairly simple to answer. Using John Imming’s Universal Blackjack Engine software, I could simulate more than 100 million hands of blackjack per day in my basement.
So, I set up a test of three Hi-Lo variations—Wong’s, Braun’s, and my own (developed via the 'Algebraic Approximation' method). I ran off 500 million hands of each strategy with a flat bet in single-deck games with Vegas Strip rules, using all indices between –15 and +15. This simulation comparison, which totaled 1.5 billion hands, may have been the lengthiest computer simulation of casino blackjack ever attempted for the purpose of answering a single question.
At the end of the test, to my surprise, there was no mathematically significant difference between any of the results.
These were the results:
Blackjack Full Indices Definition
Wong Hi-Lo: +0.477%
Snyder Hi-Lo: +0.462%
Braun Hi-Lo: +0.461%
The difference between the best win rate (Wong’s) and the worst (Braun’s) is about one sixtieth of one percent, which is not mathematically significant with only 500 million hands. It took my computer two weeks, running 24 hours per day, to run these 1.5 billion hands, and I’ll be damned if I’m going to waste any more computer time attempting to answer this question. There is virtually no dollar and cents difference to the player.
Because there are many differences among the recommended indices for these three systems, however, my simulation results led me to hypothesize that strategy index numbers may not be such precise indicators of when to alter basic strategy, or, at least, that the 'borderline' for the coin-toss decisions may be a fairly wide line.
So I followed up that column, and this initial set of simulations, with another test to see just how wide that borderline might be.
I set up a 6-deck Atlantic City game and ran off 200-million hands using Wong’s Professional Blackjack indices for this game. I used the top 18 indices.
For the second simulation, I converted each of Wong’s indices to –1, +1, or +4. I did this systematically. If Wong’s index was –1 or –2, I made it –1. I his index was 0, +1, or +2, I made it +1. His +3, +4, and +5 indices all became +4. I then ran off another 200-million hands testing this simplified version of Wong’s strategy. In both simulations, I used a 1-to-8 spread, and I also tested the effect of not betting on negative counts.
These were the results:
Wong Play All: +0.50%
Simplified Play All: +0.51%
Wong No Neg.: +0.98%
Simplified No Neg.: +0.99%
The differences between Wong’s system and the simplified version are not mathematically significant. What is meaningful for players is that a highly simplified version of the Hi-Lo strategy indice charts performs with equal power to the precise version. To the player who might find it difficult to memorize and utilize many different strategy indices, this opens the possibility of learning just three strategy indices, and learning the changes in blocks.
First you learn the few changes that occur at +1; then you learn to +5 block of changes; finally, the –1 changes. Forget the charts and flash cards with different numbers for each decision. They are a waste of time and effort.
But, how well would this approach work in single-deck games, where playing strategy is so much more important?
I set up a Reno one-deck simulation and used 60 indices from page 107 of Wong’s Professional Blackjack. I ran 100 million hands and tallied the results with both a flat bet and a 1-to-4 spread. The penetration was 75%.
I then tested two 'lite' versions of the Hi-Lo. For the first lite version, I 'widened the border,' converting Wong’s indices to either –5, -1, +1, +5 or +10. I ran off 100 million hands with this highly simplified strategy, keeping all other conditions identical.
Then, I removed 21 of the pair split indices (all but the ten splits), so that this lightest lite version not only had simplified strategy tables, but also used only 39 strategy indices as opposed to Wong’s 60.

These were my results:
Wong Flat Bet: -0.06%
Wong 1-to-4 Spread: +1.32%
Lite (60 Indices) Flat Bet: -0.05%
Lite (60 Indices) 1-to-4 Spread: +1.33%
Lite (39 Indices) Flat Bet: -0.08%
Lite (39 Indices) 1-to-4 Spread: +1.29%
The fact that the Hi-Lo Lite (60 Indices) system outperformed Wong’s by +0.01%, again, is not significant in a test of 100 million hands. The removal of the 21 pair split indices shows a bigger effect than just simplifying the indices, but even this difference is just barely on the edge of mathematical significance.
From the practical, dollars and cents perspective, it doesn’t matter which of these systems you use. These simulation results indicate that you may use a vastly simplified Hi-Lo Strategy and maintain full power, even in a one-deck game!
What does this discovery mean to card counters? It means that learning and utilizing strategy indices for any system need not be the chore that it has been. Instead of memorizing a different index number of each individual decision, you may simplify the indices using the same methodology that I did, and learn your changes in blocks.
How to Use the Hi-Lo Lite Card Counting System

Blackjack Full Indices Meaning
Here’s how to do it:
1) Learn basic strategy.
2) Learn all of the strategy changes that occur at +1. Don’t learn any other changes until you’ve mastered this block.
3) Learn the block of changes that occur at +5. Ditto.
4) Learn the blocks of changes that occur at –1; then –5; then +10, as above.
At the blackjack tables, using this simplified strategy is a piece of cake in comparison to the traditional methods of strategy variation. It’s still not as simple as the Red Seven Count, but this 'lite' (rounded) strategy retains full system power, which the Red Seven does not.
The true counts where each new block of changes kicks in are far apart. You don’t have to worry about whether the true count is +1 or +2 or +3, since you’re simply going to use your +1 block of changes until the true count gets all the way up to +5. All of the nitpicking and much of the brainwork is eliminated.
I would also again advise players, as I first advised back in 1980, to throw out your pair splitting indices.
The 0.03% (three hundredths of a percent) difference between the Hi-Lo Lite (39) and Wong’s Hi-Lo, using the 1-to-4 spread, even if real, would make very little practical dollar and cents difference to a player. Also, note that this difference is entirely due to eliminating the pair split indices, not the 'lite' approach. A player who didn’t want to give up those few hundredths of a percent could simply add back in the Lite pair split indices.
Before you go to that trouble, however, consider exactly what an 0.03% difference will mean to your expectation. A card counter using Wong’s Hi-Lo, with the full set of pair split indices, and $50 average bets, with 100 hands per hour and a 1-to-4 spread, has an expectation of about $66 per hour. The High-Low Lite, with 21 of the pair split indices removed, has an expectation of about $64.50 per hour.
Blackjack Full Indices 2020
You may also use these same indices for multiple-deck games, though you may throw out the +10 block (since you’ll rarely encounter true counts this high); and throw out the –5 block if you’re table hopping to avoid negative decks.
Here’s the Hi-Lo Lite (39) strategy I used in my simulation. Alter basic on each decision as your true count hits each block.
+1 Block:
Insurance
16 v. 10
12 v. 3 and 4
+5 Block:
16 v. 9 and A
15 v. 10 and A
12 v. 2
10 v. 10 and A
A-9 v. 5 and 6
X-X v. 5 and 6
-1 Block:
13 v. 2 and 3
12 v. 5
11 v. A
10 v. 9
-5 Block:
17 v. A
15 v. 2
14 v. 2 and 3
13 v. 4 and 5
12 v. 6
11 v. 8, 9, and 10
10 v. 8
Full Indexes Blackjack
+10 Block:
16 v. 8
15 v. 9
14 v. A
A-9 v. 2, 3, and 4
X-X v. 3 and 4
| 3 | 4 | 5 | 6 | 7 | 8 | 9 | X | A |
|---|---|---|---|---|---|---|---|---|
| 17 | -5 | |||||||
| 16 | 10 | 5 | 1 | 5 | ||||
| 15 | -2 | 10 | 5 | 5 | ||||
| 14 | -5 | -5 | 10 | |||||
| 13 | -1 | -1 | -5 | -5 | ||||
| 12 | 5 | 1 | 1 | -1 | -5 | |||
| 11 | -5 | -5 | -5 | -1 | ||||
| 10 | -5 | -1 | 5 | 5 | ||||
| A9 | 10 | 10 | 10 | 5 | 5 | |||
| XX | 10 | 10 | 5 | 5 | -5 | |||
| Ins: 1 |
Blackjack Full Indices Vs
For an expanded, updated version of the Hi-Lo Lite Card Counting System, as well as the Zen count and information on how professional gamblers win at blackjack without card counting, seeBlackbelt in Blackjack by Arnold Snyder. For the original Hi-Lo count, see Stanford Wong's Professional Blackjack. ♠